Mathematics and the Natural Sciences

. . . This world’s no blot for us, Nor blank; it means intensely, and means good: To find its meaning is my meat and drink. —Robert Browning1

I. Predictions and Unforeseen Applications

The second question, hard to answer from the standpoint of mathematics-as-art or human invention, could be posed by Morris Kline:

the study of mathematics and its contributions to the sciences exposes a deep question. Mathematics is manmade. The concepts, the broad ideas, the logical standards and methods of reasoning, and the ideals which have been steadfastly pursued for over two thousand years were fashioned by human beings. Yet with this product of his fallible mind man has surveyed spaces too vast for his imagination to encompass; he has predicted and shown how to control radio waves which none of our senses can perceive; and he has discovered particles too small to be seen with the most powerful microscope. Cold symbols and formulas completely at the disposition of man have enabled him to secure a portentous grip on the universe. Some explication of this marvelous power is called for.2

Kline is echoing a plea which has surfaced continually through history and is still waiting to be answered by those who believe that mathematics is strictly a human endeavor. When one reads articles like Nobel Prize winner Eugene Wigner’s, “The Unreasonable Effectiveness of Mathematics in the Natural Sciences,” one senses from the title Wigner’s bewilderment over the fact that purely cerebral “inventions,” the “free creations of the human mind,” have such powerful applications to the physical world. According to Wigner, “the enormous usefulness of mathematics in the natural sciences is something bordering on the mysterious and . . . there is no rational explanation for it.”3 Referring to the appearance of complex numbers in the laws of quantum mechanics, he observes: “It is difficult to avoid the impression that a miracle confronts us here . . .”4 Other prominent mathematicians agree. The brilliant John von Neumann referred to the relationship of mathematics to the natural sciences as “quite peculiar,” even though he claimed it was the “most vitally characteristic fact about mathematics.”5 There is no doubt von Neumann’s assessment is accurate. Mathematics discovered purely by thinking has powerful physical applications, nearly always unforeseen at the time of discovery.

Discoveries Applied Later

The great British scientist, Lord Kelvin, was a brilliant physicist but a poor judge of mathematical truth or usefulness. He evidently ignored the fact that

. . . by and large it is uniformly true in mathematics that there is a time lapse between a mathematical discovery and a moment when it is useful; and that this lapse of time can be anything from thirty to a hundred years, in some cases even more; and that the whole system seems to function without any direction, without any reference to usefulness, and without any desire to do the things which are useful.6

This is certainly true of quaternions and vectors, discovered in the 19th century by Sir William Hamilton. “Today nearly all branches of classical and modern physics are represented using the language of vectors. Vectors are also used with increasing frequency in the social and biological sciences.”7 But Lord Kelvin’s evaluation at the end of the 19th century was: “[Quaternions] although beautifully ingenious, have been an unmixed evil to those who have touched them in any way . . . vectors . . . have been never of the slightest use to any creature.”8

But what if mathematics is God’s thought in the creation?

In the person of his illustrious colleague, Hermann von Helmholtz, Lord Kelvin found support for another monumental gaffe. James Clerk Maxwell, in modifying Ampere’s Law, found that his additions did no violence to the mathematics of the situation, and observed that there was probably a physical phenomenon for which the new mathematical quantity was a model.

He called this a ‘displacement current,’ and he even suggested a way of producing such currents in the laboratory. Now this seemed to smack of science fiction, and the most distinguished mathematical physicists of the day (von Helmholtz and Kelvin) dismissed it as such. However, 23 years later (and this was ten years after Maxwell’s death) the German physicist Heinrich Hertz decided to see if such waves could be generated in the manner Maxwell had proposed.

The result is now history; Hertz’s experiments indeed demonstrated the reality of Maxwell’s seeming ‘science fiction,’ and the foundation of much of our communication, navigation and entertainment industries was laid . . . Trying to give physical meaning to a mathematical term had the consequence of leading to the invention, by Maxwell, of a physical concept the existence of whose physical counterpart (radio waves) was not discovered until 23 years later by Hertz.9

It is interesting that Wilder refers to the idea as an “invention,” but calls its “physical counterpart” a “discovery.” Kline hated to grant even this much. “Radio waves,” he said, “whose physical nature are still not understood, were discovered, it might almost be said invented, because mathematical reasoning demanded their existence.”10 (Italics his)

To claim that radio waves “might almost be said invented” skirts the boundaries of rational thought, yet it is indicative of the ambivalence many mathematicians bring to the study of natural law. Ernst Mach, for example, insisted that “these mental expedients have nothing whatever to do with the phenomenon itself.” Yet he admitted that “our [mathematical] conceptions of electricity fit in at once with the electrical phenomena.”11

Earlier in Mathematics in Western Culture, Kline cites another discovery which for 2,000 years was labeled “the unprofitable amusement of a speculative brain.” However, this “amusement”—conic sections— “ultimately made possible modern astronomy, the theory of projectile motion, and the law of universal gravitation.”12

In Men of Mathematics, Dr. Bell includes his example of

. . . a phenomenon of frequent occurrence in the history of mathematics: the necessary mathematical tools for scientific applications have often been invented decades before the science to which the mathematics is the key was imagined. The bizarre rule of ‘multiplication’ for matrices, . . . seems about as far from anything of scientific or practical use as anything could possibly be. Yet 67 years after Cayley invented it, [actually Cayley generalized an algebra “invented” by Hamilton] Heisenberg in 1925 recognized in the algebra of matrices exactly the tool which he needed for his revolutionary work in quantum mechanics.13

Yet Bell patronizes those who would dare mention “God” and “mathematics” in the same breath. Searching for the forest while lost in the trees, he cites other instances of the “peculiar duplicity” between mathematics and nature, but offers no explanation as to why “ . . . the by-products of these apparently useless investigations amply repay those who undertake them by suggesting numerous powerful methods applicable to other fields of mathematics having direct contact with the physical universe.”14

Speaking of probability, for instance, Bell says,

The humble origin of this extremely useful mathematical theory is typical of many: some apparently trivial problem, first solved perhaps out of idle curiosity, leads to profound generalizations which . . . may cause us to revise our whole conception of the physical universe . . . ”15

As to why many “artificially prepared” problems motivated by investigating analytic functions of a complex variable “have proven of the greatest service in aerodynamics and other practical applications of the theory of fluid motion,”16 the best Bell can come up with is “curious.” Curious, indeed, that this theory “developed without any chance of immediate use”17 from studies in mathematics for its own sake should today be used in automobile and aircraft manufacture to guarantee the streamline form having the least wind resistance.

But what if mathematics is God’s thought in the creation? Then it would be “curious” if man, created in God’s image, did not discover it by thinking, and could not use it to “subdue and replenish” the earth and take dominion over it, as God commands. When mentioning the late-breaking, powerful, unforeseen applications to physics of such mathematics as Hermitian forms and substitution groups, Bell credits Hermite and Cauchy18 respectively with the invention of these concepts. Did these men, great as they were, invent nature? Surely, “discovered” would be the more realistic label.

Earlier, in Men of Mathematics, Bell essentially admits he is using “invention” when “discovery” is the more accurate designation. He writes,

the mere spectator of mathematical history is soon overwhelmed by the appalling mass of mathematical inventions that still maintain their vitality and importance for scientific work, as discoveries of the past in any other field of scientific endeavor do not, after centuries and tens of centuries.19 (Emphasis mine)

When Gauss laid the foundation for Riemannian generalization of geometry, he may have looked upon it as his creation, his property, since its importance in physical science was not recognized for decades.20 But what of continued fractions, which “he had developed as a young man to satisfy his curiosity in the theory of numbers?” Gauss himself, a great scientist as well as mathematician, eventually applied this “purely abstract technique” to dioptics, especially lens systems.21

With a boldness surpassing even Maxwell’s, Einstein accurately predicted the gravitational deflection of light and also the red shift of spectrum under certain conditions. Through mathematics, both men prophesied “totally unknown and unforeseen phenomena” and “amplified their qualitative foresight by precise quantitative predictions which . . . were verified experimentally.”22 For Bell, the experimental verification of these prophecies “precluded any charge of mere guessing.”23

These facts hardly support the mathematics-is-art theory held by many and typified by John W.N. Sullivan:

Mathematics, as much as music or any other art, is one means by which we rise to a complete self-consciousness. The significance of mathematics resides precisely in the fact that it is an art; by informing us of the nature of our own minds it informs us of much that depends on our minds. It does not enable us to explore some remote region of the eternally existent; it helps to show us how far what exists depends on the way we exist. We are the lawgivers of the universe; it is even possible that we can experience nothing but what we have created, and that the greatest of our mathematical creations is the universe itself.24

“Mathematics,” he continued, “is of profound significance in the universe, not because it exhibits principles we obey, but because it exhibits principles that we impose.”25 This is Sullivan’s attempt to answer Kline’s deep question, of which Sullivan, parroting Kant, had his own version: “Why the external should obey the laws of logic, why, in fact, science should be possible, is not at all an easy question to answer.”26

It evidently was too difficult for Sullivan to answer, if the best he can do is make the unsupported claim that “mathematicians are the law-givers of the universe” and that the universe itself is “the greatest of our mathematical creations.” Though it fails as an answer, a better statement of the Faith of Religious Humanism would be hard to find. One is reminded of the caption on the postage stamp commemorating the Apollo-Soyuz space mission: “Man is his own star.”

Sullivan is reiterating an earlier theme of his in which he maintains,

If [the mathematician] can find, in experience, sets of entities which obey the same logical scheme as his mathematical entities, then he has applied his mathematics to the external world; he has created a branch of science.27

Sullivan’s faith-construct, emulated by many, is defective; its consequences do not fit the facts. Maxwell and Einstein did not shape the universe to fit their mathematical discoveries. Instead, they predicted quantitatively what until then were “totally unknown and unforeseen phenomena” which would be discovered in the future by others. Even Sullivan, in a different Aspects of Science, published in 1927, was impressed with the “inevitability” (italics his) of mathematical demonstrations.28 He confessed also that “ . . . mathematics . . . has repeatedly shown itself applicable to real happenings, however little notions of utility may have played a part . . . in its creation.”29

Crystallography provides other instances of what James R. Newman called “mathematical prevision.”30 For example, Hamilton “predicted mathematically that a wholly unexpected phenomenon,” namely, a cone of infinitely many rays of refracted light, would be found in connection with the refraction of light in biaxial crystals.31 Newman also claims that

mathematicians . . . decreed the permissible variations of internal structure of crystals before observers were able to discover their actual structure. Mathematics, in other words, not only enunciated the applicable physical laws, but provided an invaluable syllabus of research to guide future experimenters.32

Harvard professor Phillipe Le Corbeiller, in an article worth reading in its entirety, gives an example: “Mathematicians have proved that the symmetry elements of crystals can be grouped in 32 different ways, and no others. Crystallographers have classified every known crystal into one of these 32 crystal classes.”33 Would Sullivan have us believe the mathematicians created crystals because they predicted their structure precisely?

Then there is the phenomenon of Gauss, Hamilton, Riemann, Elwin Bruno, Christoffel, and Hilbert, working independently on parts of what turned out to be the same esoteric geometry, under the delusion not only that they were creating unique works of art, but also that their efforts had no usefulness beyond aesthetics.34 As Carl G. Hempel writes,

The geometrical theory which is used to describe the structure of the physical universe is of a type that may be characterized as a generalization of elliptic geometry. It was originally constructed by Riemann as a purely mathematical theory, without any concrete possibility of practical application at hand. When Einstein, in developing his general theory of relativity, looked for an appropriate mathematical theory to deal with the structure of physical space, he found in Riemann’s abstract system the conceptual tool he needed. This fact throws an interesting sidelight on the importance of scientific progress of that type of investigation which the ‘practical-minded’ man in the street tends to dismiss as useless, abstract mathematical speculation.35

When it comes to the relation of mathematics to the physical sciences, the judgment of mathematicians, even on their own discoveries, is often no better than that of the “man in the street.” G.H. Hardy , the militant “pure” mathematician who regarded God as his personal enemy,36 boasted, “I have never done anything ‘useful’. No discovery of mine has made, or is likely to make, directly or indirectly, for good or ill, the least difference to the amenity of the world.”37 New parents whose baby suffers from haemolytic disease would bless Hardy while disagreeing with his evaluation of his work. A principle he established (to which he attached “little weight”) is “of central importance in the study of Rh-blood groups and the treatment of [this] disease of the newborn.”38

Also, Hardy was fascinated by Riemann’s Zeta function, which deals with the number of primes less than a given number. Never in the far reaches of imagination would Hardy or anyone else connect this esoteric concept in number theory with something so mundane as the study of furnace temperatures, but such is its application today.39

Kline at times denigrates modern “pure” mathematics. He quotes, for example, chemist Josiah Willard Gibbs’ remark that “the pure mathematician can do what he pleases but the applied mathematician must be at least partially sane.”40 Kline cannot, however, escape the fact that mathematical concepts “not drawn directly from nature” and even seemingly “at variance with nature,” with “no reason” for anyone to look for their applications to physics, work “marvelously well.”41

He goes on to say that

the application made in the 19th and 20th centuries [of ] purely mathematical constructs are even more powerful and marvelous than those made earlier when mathematicians operated with concepts suggested directly by physical happenings.42

This is history for which Kline, labeling as “farfetched” belief in a mathematically designed universe, cannot account. “Why,” he asks plaintively, “should long chains of pure reasoning produce such remarkably applicable conclusions?” Kline’s confession is all that is left him: “This is the greatest paradox in mathematics.”43

Speaking of “paradoxes,” I wonder what Kline thought of Alonzo Church’s calculus of lambda conversion, which David Berlinski says “at first seems pointlessly complex and pointlessly abstract.” Many years later, it “turned out to be instrumental in the development of various computer languages.” The amazing thing is that Berlinski clings to his assumption that “using . . . his own powerful imagination, Church proceeded to create a universe out of thin air,” and is forced to refer to it as “another queer and troubling example of pure thought preceding its instantiation in matter.”

The Christian recognizes it as another example of the truth of Hebrews 1:3, transcendent thought upholding the universe, which Church is discovering, not creating. Berlinski, despite his humanistic assumptions about the origin of mathematics, sounding quite biblical, calls Church’s work “an opening into a world beyond the world of symbols.”

Two final examples, which John von Neumann calls “strange,” of pure mathematical thought having unexpected scientific applications

are given by differential geometry and group theory: they were certainly conceived as abstract, nonapplied disciplines and almost always cultivated in this spirit. After a decade in one case, and a century in the other, they turned out to be very useful in physics. And they are still mostly pursued in the indicated, abstract, nonapplied spirit.44

A statement by Alfred North Whitehead epitomizes the preceding observations. To Whitehead,

Nothing is more impressive than the fact that as mathematics withdrew increasingly into the upper regions of ever greater extremes of abstract thought, it returned back to earth with a corresponding growth of importance for the analysis of concrete fact . . . the paradox is now fully established that the utmost abstractions are the true weapons with which to control our thought of concrete fact.45

Bourbaki clearly implies that the nature-mathematics marriage is impossible to explain if mathematics is considered an invention of man’s mind.

That there is an intimate connection between experimental phenomena and mathematical structures, seems to be fully confirmed in the most unexpected manner by the recent discoveries of contemporary physics. But we are completely ignorant as to the underlying reasons for this fact.46

Leaving no doubt about “their” ignorance, Bourbaki goes on to say,

From the axiomatic point of view, mathematics appears thus as a storehouse of abstract forms—the mathematical structures; and it so happens—without our knowing why—that certain aspects of empirical reality fit themselves into these forms, as if through a kind of preadaptation.47

If mathematics is the thinking of the Creator of “empirical reality,” “preadaptation” is exactly what would be expected. Bourbaki’s ignorance stems from their assumption either that no personal-infinite God is there, or if He is there, it does not matter.48

II. Abstractions, Models, and Applications

F.E. Browder of the University of Chicago, writing in The American Mathematical Monthly, speaks “not about Mathematics simple, but Mathematics I (counting, measuring, calculating), Mathematics II (applications to other disciplines), Mathematics III (research or “pure” mathematics), and Mathematics IV . . . the transcendent ideal of mathematics as a fundamental and universal form of knowledge.”49

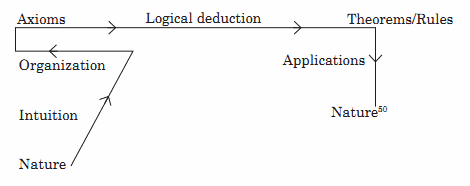
Deducing the mathematical models, the ideas, from natural clues is called “abstracting.” Carl Allendoerfer presented this process schematically:50

Attempting to define “what is meant by calling mathematics an ‘abstract science,’” Whitehead points out that

the first noticeable fact about arithmetic is that it applies to everything, to tastes and to sounds, to apples and to angels, to the ideas of the mind and to the bones of the body. The nature of the things is perfectly indifferent, of all things it is true that two and two make four. Thus we write down as the leading characteristic of mathematics that it deals with properties and ideas which are applicable to things just because they are things, and apart from any particular feelings, or emotions, or sensations, in any way connected with them.51

Whitehead also claimed “all science as it grows . . . becomes mathematical in its ideas.”52

A specific example of abstracting is provided by the swinging pendulum as it slowly loses energy through friction and finally comes to rest. The scientist by trial and error can produce a formula which approximates the laws of motion which the Bible claims God spoke into existence. Depending upon how accurate the scientist’s instruments of measure, how clear his eyesight, and how closely the actual situation is modeled by his formula, he can use it to predict results approximately. Some have called this type of abstraction “descriptive” or “experimental” mathematics.

Here the matter would rest if it were not for the mathematician making his entrance stage right, asking his ubiquitous, “What if?” “What if,” questions he, “we ignore air resistance and other friction, ignore also temperature and so on, and conceptualize an ideal pendulum under ideal conditions? What equations would describe this situation?”

Now he is abstracting from the physical, tuning in to the thinking behind the word of creation and consistence. Many non-mathematicians, some scientists, and even a few mathematicians, have ridiculed abstract pictures as being valueless, because the mathematical model is more precise than its physical counterpart. So far as usefulness goes, they would have been content to stop at the descriptive level, relegating “pure” mathematics to the world of art or games.

It is now clear, however, that the discoveries which turn out to have the most empirical power occur in the rarified atmosphere of pure mathematics, free from the limitations of imprecise measuring instruments and the inability to use them precisely. As Bell points out, “The problem of finding the mathematical expressions for the intrinsic laws of nature is replaced by an attackable one in the theory of invariants.”53

Back at the pendulum, the mathematician begins stringing together “what-ifs” and he must be watched, for he is like a lover; “grant a mathematician the least principle and he will draw from it a consequence which you must grant him also, and from this consequence another.”54 Finally, a description of natural laws is produced which governs a wider collection of physical phenomena than just pendular motion. Those who deprecate the value of time spent trying to discover the idea-models behind nature are dumbfounded when the mathematician introduces them to a scenario which applies more widely and powerfully, though still approximately, to the workings of creation.

James R. Newman puts it this way,

Modern mechanics describes quite well how real bodies behave in the real world; its principles and laws are derived, however, from a nonexistent conceptual world of pure, clean, empty, boundless Euclidean space, in which perfect geometrical bodies execute perfect geometrical figures.55

He goes on to say,

Until the great thinkers, operating, in Butterfields’s words, ‘on the margin of contemporary thought,’ were able to establish the mathematical hypothesis of this ideal platonic world, and to draw their mathematical consequences, it was impossible for them to construct a rational science of mechanics applicable to the physical world of experience.56

Because it is a conceptual world, Newman is led to the erroneous conclusion that it is “nonexistent” and “platonic.” Otherwise, he could be describing God’s creation thought and its connection to “real bodies” and “the physical world of experience.”

One of the first to study the motion of the pendulum in this way was Galileo, who thought that

mathematical abstractions got their validity as statements about Nature by being solutions of particular physical problems. By using this method of abstracting from immediate and direct experience, and by correlating observed events by means of mathematical relations which could not themselves be observed, he was led to experiments of which he could not have thought in terms of the old commonplace empiricism.57

Another example of the process and value of abstracting mathematics from the physical is found in vector, or linear, algebra. It began in the engineering representation of a force as an arrow drawn on paper, aimed a certain way to indicate the direction of the force, and of a certain length to describe its magnitude. In other words, a vector was a scale model of the size and direction of a force. Then, with ruler and protractor, engineers could approximate the sum of forces acting on a body, or separate a force into an equivalent system of forces.

“What if?” the mathematician interrupts again.

What if we assume we know the exact measures of these angles of direction, and lengths of the arrows? What if we replace the drawing of an arrow with an ordered pair of numbers, the first representing the distance the arrow travels horizontally, and the second, the distance it travels vertically? What if we could define an addition operation on these pairs which would correspond to the ‘addition’ of arrows by scaling the drawing? Could we find an identity, and would there be inverses?”

He exits stage left and behind the scenes discovers an algebra not only for two or three dimensions, but for n-dimensional space. Between acts, he catches the engineer backstage and hands him the script to a vastly superior, more powerful understanding of processes and products of what I believe to be God’s creative imagination. In fact, center stage—the laboratory—linear algebra, or tensor analysis, turns out to be the most general picture the scientist yet possesses of the universe.

Dr. Alfred Inselberg of the I.B.M. Los Angeles Scientific Center no doubt utilized linear algebra in his construction of a mathematical model of the human ear. Explains Dr. Inselberg,

We can generate a computer model, based on the mathematical model, that describes the behavior of the cochlea. We can then do various experiments on the computer that could not be done on the actual ear. We can then use the computer to discover what sort of physical defect results in a particular hearing impairment. Since the defect can be ‘repaired’ mathematically in the model, we could, in some cases, even suggest appropriate treatment.58

About his research Dr. Inselberg says, “We must remember that no mathematical model can be as precise as the real thing. But our model is answering questions that couldn’t be answered without it.”59 It is true that no man-made model is as precise as the real thing. But I think in God’s mind rests the precise mathematical description of each individual’s ear, just as He knows the exact mathematics of each snowflake. Man is allowed to discover the hexagonics which, in general, describe snowflakes, and can introduce modification to approximate the characteristics of individual flakes. Dr. Inselberg is involved in a similar process with the ear, only multi-dimensionally. Cassius Jackson Keyser was correct when he stated, “Mathematics, even in its present and most abstract estate, is not detached from life. It is just the ideal handling of the problems of life . . . ”60

It is true that some mathematicians spend their lives making discoveries in mathematic’s “most abstract estate” without knowing the natural or creational base from which it springs, and worrying neither about that nor about applications to the creation of what they are doing. R.L. Wilder’s judgment of such:

I have often observed that among the most capable, research-wise, of new Ph.D.’s, can often be found the greatest lack of knowledge concerning the background and significance of their work, as well as abysmal ignorance of the reasons for doing it and of the general nature of mathematics. In short, they are uneducated specialists. If you ask them why they are specialists, the best reason they can give is that this is the way to get results which merit publication and hence a good job.”61

If what a mathematician discovers is really true, it will be consistent with previously discovered mathematics which has passed time’s test, and it will be traceable to natural scientific origins or applicable to the creation. As we have seen, sometimes there is a considerable waiting period until the horizons of mathematical discovery widen so that apparently separate, or even contradictory, systems can at last be seen dwelling harmoniously under a bigger sky.

The great von Neumann proffered some cogent observations on this subject. “I think that it is a relatively good approximation to truth,” he said,

that mathematical ideas originate in empirics, although the genealogy is sometimes long and obscure. But, once they are so conceived, the subject begins to live a peculiar life of its own and is better compared to a creative one, governed by almost entirely aesthetic motivations, than to anything else and, in particular, to an empirical science. There is, however, a further point which . . . needs stressing. As a mathematical discipline travels far from its empirical source . . . only indirectly inspired by ideas coming from ‘reality,’ . . . It becomes . . . purely l’art pour l’art. . . . there is a grave danger that . . . the stream, so far from its source, will separate into a multitude of insignificant branches and . . . become a disorganized mass of details and complexities. . . . after much ‘abstract’ inbreeding, a mathematical subject is in danger of degeneration. . . . whenever this stage is reached, the only remedy seems to me to be the rejuvenating return to the source: the reinjection of . . . directly empirical ideas. I am convinced that this was . . . necessary . . . to conserve the freshness and vitality of the subject . . .62

Far from mistaking mathematics to be purely art, the ancient Greeks “viewed mathematics as an in depth study of physical phenomena.” In fact, the root word, mathema (μάθημα), means “that which is learned,” as opposed to “that which is produced” or “that which is imposed.”63

Drs. Kline and Bell are critical of mathematicians and textbook writers who ignore or downplay the processes of abstracting mathematics from nature or applying mathematics to nature. Kline decries the fact that “the relationship of mathematics to the study of nature is not presented in our dry and technique-soaked textbooks,” and that “mathematics is valuable primarily because of its contributions to the understanding and mastery of nature has been lost sight of by some mathematicians who wish to isolate the subject and offer only an eclectic study.”64 Kline maintains “an undue emphasis on abstraction, generality, and logically perfect deductive structures has caused mathematicians to overlook the real importance of the subject.”65

Caution about becoming lost in logic to the exclusion of experience is a recurring theme of Kline’s. Discussing the development of mathematics, he insists “it was not the logic . . . but arguments by analogy, the physical meaning of some concepts, and the obtainment of correct scientific results”66 which determined correct directions. “Logic,” Kline says, “does not dictate the contents of mathematics, the uses determine the logical structure.”67 For Kline, “Logic may be a standard and an obligation of mathematics, but it is not the essence. It is the uses to which mathematics is put that tell us what is correct.”68

Kline maintains this theme in a book published in 1980. “What preserved the life of mathematics,” Kline insists,

was the powerful medicine it had itself concocted—the enormous achievements in celestial mechanics, acoustics, hydrodynamics, optics, electromagnetic theory, and engineering—and the incredible accuracy of its predictions. There had to be some essential—perhaps magical—power in a subject which, though it had fought under the invincible banner of truth, has actually achieved its victories through some inner mysterious strength.69

Both before and after this assertion, Kline states his philosophical standpoint. “Mathematicians had given up God and so it behooved them to accept man. . . . Nature’s laws are man’s creation. We, not God, are the lawgivers of the universe. A law of nature is man’s description and not God’s prescription.”70

Kline is willing to assign mathematics to the land of the “incredible,” the “magical,” and the “mysterious,” rather than move from this unrealistic standpoint. Therefore, to Kline, “the remarkable confirmation and power of what has been applied remains to be explained.”71

Claiming “intuition and physical arguments rather than logic guided Newton and Leibnitz along the proper paths,”72 Kline’s presuppositions about the nature of mathematics force him to label this “a very fortunate circumstance,” though it is exactly what is expected from the perspective of a Christian world-view.

“The profound study of nature is the most fecund source of mathematical discoveries. [The] fundamental elements are those which recur in all natural phenomena.”

In praising Maxwell’s electromagnetic theory which he calls “so profound and comprehensive that it beggars the imagination,” Kline admits there is “a plan and order in nature which speaks more eloquently to man than nature’s prancings.”73 So Kline evidently believes in the existence of some communicative intelligence behind nature, despite saying elsewhere that man “outgrew” the idea it was God “speaking” through mathematics. Kline’s comments on Maxwell’s ideas paraphrase those written many years previously by Heinrich Hertz, after the great scientist in his laboratory verified Maxwell’s predictions. Said Hertz, “It is impossible to study this wonderful theory without feeling as if the mathematical equations had an independent life and an intelligence of their own, as if they were wiser than ourselves, indeed wiser than their discoverer . . . ”74 The drawstring of the bag loosens to let the cat wriggle out when Kline confesses that the true value of mathematical formulas “lies in the fact that they apply to so many varied situations on heaven and earth.”75

For his part, Bell quotes Fourier as saying, “The profound study of nature is the most fecund source of mathematical discoveries. [The] fundamental elements are those which recur in all natural phenomena.”76 Bell then criticizes

pure mathematicians [who] sometimes like to imagine that all their activities are dictated by their own tastes and that the applications of science suggest nothing of interest to them. Nevertheless some of the purest of the pure drudge away their lives over differential equations that first appeared in the translation of physical situations into mathematical symbolism, and it is precisely these practically suggested equations which are at the heart of the theory.77

Neither Kline nor Bell give any place to religious or philosophical considerations when discussing the nature of mathematics. Each claims that mathematics is art, giving credit for its construction solely to man’s mind. That this assumption leads to the foregoing contradictory statements deters them not at all.

A prime example of the mathematical base of nature is given in the work of Michael Faraday, who, as Charles Sanders Peirce observes, “evolved” the mathematical ideas “requisite” to electricity by pursuing its study “resolutely, without any acquaintance with mathematics.”78 Yet, as previously stated, it is possible, even desirable, to tune in to mathematics at the thought level “and then to apply it to electricity, which was Maxwell’s way.”79

Charles’ father, Benjamin produced a definition of mathematics often misquoted as “the science drawing necessary conclusions.” As son Charles points out, this is a definition of logic. The rendering should be, “The science which draws necessary conclusions,”80 which once more fixes the jewel in its required setting of natural phenomena.

Another mathematician particularly adept at hearing and recording nature’s mathematical speech was Joseph-Louis Lagrange,

who with a pen in his hand . . . was transfigured; and from the first, his writings were elegance itself. He would set to mathematics all the little themes on physical inquiries which his friends brought him, much as Schubert would set to music any stray rhyme that took his fancy.81

Joseph Fourier began with actual music and translated it into mathematical components. He showed that “all sounds, vocal and instrumental, simple and complex, are completely describable in mathematical terms.”82 Even the “wailing of a cat . . . is no more complex mathematically than a simple trigonometric function. Those dull, abstract formulas . . . are really all around us. We give voice to them whenever we open our mouths and we hear them whenever we prick up our ears.”83

Though Gauss was the “Prince” of mathematicians, he was also one of the greatest physicists the world has known. His work in geodetic surveying no doubt appeared mundane to some of his contemporaries, yet this research enabled Gauss to develop the mathematics of conformal mapping, “which is of constant use in electrostatics, hydrodynamics and . . . the theory of the air foil.”84

Kepler and Newton saw the light at the end of the tunnel almost simultaneously when they discovered independently the amazing fact that the derivative and the integral were essentially inverses. The tunnel was “clearly empirical. Kepler’s first attempts at integration were formulated as ‘dolichmetry’—measurement of kegs—that is volumetry for bodies with curved surfaces.”85 Those whose only experience with integral calculus was in a classroom might never learn that its discovery was empirical. To them it will always remain “the area under a curve” on a piece of graph paper. As John von Neumann pointed out,

Newton invented the calculus ‘of fluxions,’ essentially for the purposes of mechanics—in fact, the two disciplines, calculus and mechanics, were developed by him more or less together. The first formulations of the calculus were not even mathematically rigorous. An inexact, semi-physical formulation was the only one available for over 150 years after Newton! And yet, some of the most important advances of analysis took place during this period, against this inexact, mathematically inadequate background! Yet no mathematician would want to exclude it from the fold—that period produced mathematics as first class as ever existed!86 (Exclamation points are von Neumann’s!)

So mathematics is uncovered in the construction of abstract models of nature, and these models always form a unity with mathematics discovered solely by intellectual pursuits. Referring to Laplace’s “equation of continuity,” Bell is astonished that this “physical platitude, when subjected to mathematical reasoning, should furnish unforeseen information which is anything but platitudinous.”87

Having gained access to Browder’s Mathematics III and IV through the portals of I and II (see page 27), mathematicians are able to trace the beautiful patterns of natural language by thought alone, without reference to the tangible, creational base of which the patterns are models. Philip Jourdain wrote, “ . . . it gradually appeared to us quite clearly that there is such a thing at all as a Mathematics—something which exists apart from its application to natural science.”88 This is the aspect of mathematics which leads some mathematicians to conclude it is a creative art, not realizing the possibility they are thinking God’s creation thought after Him.

But mathematics found by thinking always develops powerful creational applications. If this is not the case, if “the conceptual system should ever gain ascendency over actual research, or emancipate itself from the firm control of sensory experience, . . . as Einstein has said, scientific thinking must degenerate into ‘empty talk.’”89

Here is Philippe Le Corbeiller’s delineation of the mathematical process of abstraction: “In any new science,” writes he,

purely descriptive knowledge is the first stage of advance. Next comes the establishment of quantitative laws . . . In the third stage of scientific knowledge which we might call deductive or axiomatic, the natural laws obtained by observation are shown to be necessary logical consequences of a few hypotheses or assumptions.90

This sounds as if he has mathematics under control in a neat, tidy, man-made corral, with no great Mathematician needed, until one reads his next sentence: “The surprising thing about the examples of deductive knowledge which we know today is the extreme simplicity of the assumptions and how rich and far removed are their consequences.”91 And it is “astounding” to Herman Weyl, “that the laws show such a simple mathematical structure, while the quantitative distribution of the state quantities in the world continuum is incredibly complicated.”92

Not only do mathematical models solve physical problems, but the reverse is also true.

In some cases a physical experiment is the only means of determining whether a solution to a specific problem exists; once the existence of a solution has been demonstrated, it may then be possible to complete the mathematical analysis, even to move beyond the conclusions furnished by the model—a sort of bootstrap procedure. . . . ‘many of the fundamental theorems of function theory were discovered by Riemann, [merely] by thinking of simple experiments concerning the flow of electricity in thin sheets’—without even approaching the laboratory.93

Le Corbeiller’s study in crystallography leads him to similar conclusions: “So here we have geometry again, and arithmetic, but this time it does not all take place in our heads. Here nature invents the rules of the mathematical game . . . ”94

Christians believe the Mind partially revealed behind mathematics to be God’s. For others, it is a “terra incognita” leading to contentless mysticism. An example is given by the numbers 1,2,3,5,8,13,21, . . . , discovered in the thirteenth century by the Italian, Fibonacci. To produce a member of the sequence, you add the preceding two numbers. These numbers are encountered not only by mathematicians and scientists, but also by artists, musicians and architects. The ratio of two successive numbers in the sequence, after the 14th, is .618034 to 1. Since the Greeks practically worshiped this fraction, calling it the “golden mean,” those may be excused who think its continual reoccurrence in art and architecture is by design and not because it is “naturally” the most pleasing to the eye. What then motivates writers like William Hoffer to refer to the Fibonacci quotient as being “part of a mystical natural harmony?”95 The answer lies in the fact that this mysterious ratio is also “intimately connected with the strange twists of nature,” non-human entities like sunflowers, snail shells, and “the great spiral galaxies of outer space.”96

An example is given by the numbers 1,2,3,5,8,13,21, . . . , discovered in the thirteenth century by the Italian, Fibonacci. . . . These numbers are encountered not only by mathematicians and scientists, but also by artists, musicians and architects.

The intimate connection to which Hoffer refers is the equiangular or logarithmic spiral, which was discovered by Jacob Bernoulli and whose construction is based on “golden mean” rectangles. Christians and “mysticists” both recognize, “It is not in [nature], but in Something far beyond her, that all lines meet and all contrasts are explained.”97

Why Is Mathematics in Nature

Even Einstein was puzzled. “How can it be,” he asked, “that mathematics, being after all a product of human thought . . . independent of experience, is so admirably appropriate to the objects of reality?” Einstein’s “answer,” often quoted, only muddies the water: “As far as the laws of mathematics refer to reality, they are not certain; and as far as they are certain, they do not refer to reality.”98 The Christian would say, “Perhaps, Dr. Einstein, the problem lies in your assumption that mathematics is ‘a product of human thought.’ If mathematics really is God’s thought, He being the Creator and Sustainer of the ‘objects of reality,’ the answer to your question is clear.”

Morris Kline rejects the theistic view of the origin of mathematics as “a popular [?] fallacy most difficult to dislodge.” Since Kline’s assumptions regarding the nature of mathematics are similar to Einstein’s, his arrival at a similar philosophical dead-end is not unexpected. “Though devoid of truth,” says he, “mathematics has given man miraculous power over nature,” which Kline calls the “greatest paradox in human thought.”99 He devotes many pages of Mathematics in Western Culture to an attempt to resolve this “paradox,” failing spectacularly.

In a 1981 magazine interview he admitted, “Today there is no agreement among mathematicians on fundamental principles . . . the situation is a muddle. In the end, we just don’t know why mathematics works as it does. We’re faced with a mystery.”100 This is a strange conclusion if mathematics is, as Kline insists, “a man-made, artificial subject.”101 In 1981, Kline was still maintaining “it is not the truth,” and still confessing, “nevertheless it can make rather remarkably accurate predictions about physical phenomena.”102 As we have seen, “remarkably accurate” is an understatement.

Evolutionism

Nothing could be more damaging to the theory of evolution than the fact that God orders the universe mathematically. Evolutionists reject out of hand Kepler’s claim that “the chief aim of investigations of the external world should be to discover the rational order and harmony which has been imposed on it by God and which He revealed to us in the language of mathematics.” Evolutionism demands faith to believe that from nothing, by time plus chance plus nothing, came everything.

I once asked a professor in a biology class where the first little blob of matter came from. His answer: “We don’t think about that.” Translation: “I accept the existence of the first blob on faith.” Implication 1: Evolution is a blob based religion, and by “blob,” I include the big bang, Miller’s trapped amino acids, Guth’s “fluctuation in a false vacuum” and all the other desperate attempts by evolutionists to account for origins. Implication 2: Darwin should have called his book Post Origin of the Species.

The twin gods evolutionists worship are time and statistics. If you gag at the idea that a camel, a chrysanthemum, cerise, C-sharp, chromium, and conscience all could evolve from the same little piece of pre-existent goop, not to worry—just toss in another couple million years and let statistics work its magic.

In college we used to laugh at the definition which calls statistics the practice of drawing a mathematically precise line from an unwarranted assumption to a foregone conclusion. In the years since, however, I have come to realize that this “definition” is realistic. If a mathematician is handed a sackful of data, he/she can analyze it mathematically, extracting the statistics (mean, median, variance, etc.). But what is the point? The sample space from which the data came is affected by bias, and the results can be tweaked (lop off the outliers, apply “corrective” factors, etc.) to “prove” any preconceived notion one harbors. I’ve reached the point where the phrases “statistics show,” “studies show,” “polls show,” “research shows,” preclude me from believing anything which follows.

Harvard professor and evolutionist Richard Lewontin speaks honestly about the way a scientist’s assumptions color results, making science not quite so “pure” as its practitioners would have the public believe. “We take the side of science in spite of the patent absurdity of some of its constructs, in spite of its failure to fulfill many of its extravagant promises of health and life, in spite of the tolerance of the scientific community for unsubstantiated just-so stories, because we have a prior commitment, a commitment to materialism. It is not that the methods and institutions of science somehow compel us to accept a material explanation of the phenomenal world, but, on the contrary, that we are forced by our a priori adherence to material causes to create an apparatus of investigation and a set of concepts that produce material explanations, no matter how counterintuitive, no matter how mystifying to the uninitiated. Moreover, that materialism is an absolute, for we cannot allow a Divine Foot in the door”103 Lewontin’s disclaimer is certainly true of evolutionist “research,” the result of which might more accurately be labeled—if I might coin a word—“presult.”104

Yes, a spoonful of eons with a dash of statistics makes the random selection medicine go down, in the most deceitful way. Case in point: Stephen Jay Gould’s drunk who comes out of a bar and staggers down the sidewalk. He will bounce off the walls many times, but there is a statistical chance he may fall into the street. Therefore, evolution is true. This is “scinalogy,” scientific “proof ” by analogy.

When scientists attempt to stuff statistics into the many holes in the dike of evolutionism, their discourse on the subject is often couched in what E.L. Doctorow labeled “high-tech baroque,105 the kind of diction that is self insulating and self-ennobling . . . very often lyric and almost always metaphorical. It suggests something is there when in fact nothing is there at all.”

Summary

The relationship between mathematics and science to Kline is “mysterious” and “miraculous;” to Wigner “unreasonable;” to von Neumann, “quite peculiar;” to Bourbaki “unexpected;” to Bell it is “curious;” to Whitehead “a paradox;” and Einstein asked, “How can it be?” If the “peculiar” usefulness of mathematics in natural science cannot be rationally accounted for from the perspective of mathematics-as-art or human invention, those of this persuasion should ask themselves if their position is realistic. After all, while these mathematicians are entitled to their own points of view, they are not entitled to their own facts.